There was a great opinion piece published in the Washington Post on Sunday by Dr. Bob Wachter and Dr. Ashish K. Jha arguing that it might be time to stop stockpiling the second dose of the Pfizer and Moderna vaccines in order to give out more first doses. 

How effective would it be to give just one shot? We don’t know for sure, but, luckily, it seems that these vaccines are probably 80-90% effective after just the first dose. (Source 1, 2)

How long that protection lasts isn’t clear, but hopefully, we improve our vaccination rollout in the coming months and are able to give everyone a second dose (simply later than originally planned). 

One unknown about this approach -- What’s better for containing the spread of COVID-19: vaccinate more people with a less effective vaccine, or fewer people with a more effective vaccine?

To try to answer this, I expanded my COVID-19 model that was built to reflect some of the unique characteristics of the disease (namely, the importance of superspreaders). Link to more detail on that here.

Methods:

(note: skip this if you just want to hear the results)

I took the old model and added in the ability for individuals to be vaccinated. Because we are mostly interested in short-term effects here, all vaccinations are conducted in the first 30 days.  If, for example, we want 30% of the population to be vaccinated, 1% will be vaccinated every day for the first 30 days. The model runs for 60 days total. 

The full model is available here for you to download and play with.

Variables of note:

• Vaccine Efficacy

• Number of people receiving the vaccine

I ran the simulation with two different starting populations: one where the virus was already spreading uncontrollably, with an initial infection rate of 1 out of every 100 people, and another with a more controlled spread, starting with 1 out of every 1000 people infected. 

For each of these populations, over 30 days, between 5%-80% of the population was vaccinated. For each of these vaccination percentages, the vaccine had between 45% and 95% efficacy. For each of these combinations of settings (for example: 10% of the population vaccinated with a vaccine that is 80% effective), the model repeated the experiment 100 times (agent-based models are stochastic, leading to a broader range of outcomes for a set of starting conditions; this makes them more realistic, but also more work).

What are we measuring? At the end of each 90 day period, we’re measuring two factors: 

• what percentage of the population remains uninfected

• what was the maximum percentage of the population that was infected at one time

Together, these give a decent proxy for both the intensity and impact of the disease.

Results:

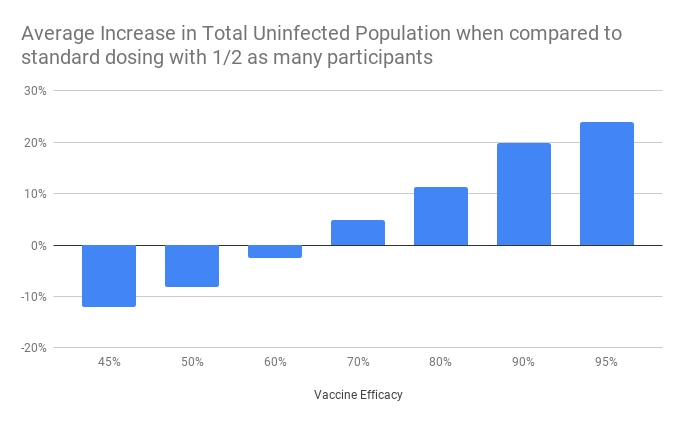
What’s important to compare here is vaccinating X number of people with the standard 2-dose vaccine with vaccinating 2X number of people with the less effective, single dose. 

To make the data more readable, let’s take the average across all vaccination cohorts:

This is assuming that the standard 2-dose vaccine has an efficacy rate of 95%. So if giving the vaccine with only 1-dose is still 95% effective, and we give it to twice as many people, then in the simulations there was a ~25% improvement in the number of uninfected people remaining in the community at the end. It’s really important to note here that this effect persists even if you’re vaccinating significantly less than 25% of the population. This increase in healthy people comes not just because they were directly protected by the vaccine, but because the vaccine was effective at slowing transmission of the virus. If you want to dive into all of the data, link is here.

We can see that there are benefits even if the vaccine is only 70% effective in its 1-dose form. Protecting more people, even with a less robust vaccine pays dividends over time. 

We see the same thing when we look at the other important metric: Max-Infected.

When we’re doubling the number of people who receive an equally efficacious vaccine, we reduce the peak of the infection wave by about 15%.

What’s interesting to note here is the break-even point. Both Max-Infected and Total Uninfected indicate that doubling the number of people receiving the vaccine is only worth it if the version you’re giving them is at least 65% effective. This is actually somewhat counterintuitive! I had assumed that if you gave twice as many people a vaccine that was half as effective, it would be better than the alternative! In fact, this doesn’t seem to be the case.

I’d speculate that this is in part because of the unique transmission dynamics of the disease, and its spread being driven by a relatively small subset of all infected people who are much more infectious than the “average” sick person. 

Conclusion:

There are risks to delaying the second dose of the vaccine. For example, the risk of selecting for antibody resistance, the likelihood of which I’m grossly unqualified to estimate, but this virologist is concerned about.

There’s also the risk that the protection provided by the first dose wears off quickly, and we’re back to square one. This seems somewhat unlikely, but again, I’m not the person to ask. 

However, I think it’s important to understand what the transmission impact would be of changing our vaccine tactics, and I think it would likely be beneficial to delay the second dose so we can more widely distribute the first dose.

The other factor that makes me more strongly in favor of the “1-dose” policy is the new Covid variant which seems to be much more contagious. Although not more deadly on an individual level, it will magnify the effect of any decisions we do or don’t make to try to slow the spread of the disease. In light of this, we ought to remember how important early and forceful interventions are when combatting exponential growth. 

Given the degree to which the federal government has been bungling this rollout (bungling is perhaps too active a verb -- “abdicating all responsibility” would be more accurate), any policy that gets more shots in more arms would seem to be beneficial at this point in time.